Astro Fisika: Apa Itu Angka Knudsen?

Saturday, 15 June 2013

Apa Itu Angka Knudsen?

Didefinisikan sebagai rasio dari rata-rata panjang jalur bebas molekular terhadap suatu skala panjang fisik representatif tertentu. Skala panjang ini dapat berupa radius suatu benda dalam suatu fluida.

Secara sederhana, angka Knudsen adalah berapa kali panjang diameter suatu partikel akan bergerak sebelum menabrak partikel lain.

Intro:

The Knudsen number (Kn) is a dimensionless number defined as the ratio of the molecular mean free path length to a representative physical length scale. This length scale could be, for example, the radius of the body in a fluid.

The number is named after Danish physicist Martin Knudsen (1871–1949). Who taught and conducted research at the Technical University of Denmark. He is primarily known for his study of molecular gas flow and the development of the Knudsen cell, which is a primary component of molecular beam epitaxy systems.

The Knudsen number is a dimensionless number defined as:

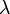$\mathit{Kn} = \frac {\lambda}{L}$

where

$\lambda$ = mean free path [L¹]
$L$ = representative physical length scale [L¹].

For an ideal gas, the mean free path may be readily calculated so that:

$\mathit{Kn} = \frac {k_B T}{\sqrt{2}\pi\sigma^2 p L}$

where

$k_B$ is the Boltzmann constant (1.3806504(24) × 10⁻²³ J/K in SI units), [M¹ L² T^-2 θ^-1]
$T$ is the thermodynamic temperature, [θ¹]
$\sigma$ is the particle hard shell diameter, [L¹]
$p$ is the total pressure, [M¹ L^-1 T^-2].

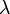For particle dynamics in the atmosphere, and assuming standard temperature and pressure, i.e. 25 °C and 1 atm, we have $\lambda$ ≈ 8 × 10⁻⁸ m.

Relationship to Mach and Reynolds numbers in gases

The Knudsen number can be related to the Mach number and the Reynolds number:
Noting the following:
Dynamic viscosity,

$\mu =\frac{1}{2}\rho \bar{c} \lambda.$

Average molecule speed (from Maxwell-Boltzmann distribution),

$\bar{c} = \sqrt{\frac{8 k_BT}{\pi m}}$

thus the mean free path,

$\lambda =\frac{\mu }{\rho }\sqrt{\frac{\pi m}{2 k_BT}}$

dividing through by L (some characteristic length) the Knudsen number is obtained:

$\frac{\lambda }{L}=\frac{\mu }{\rho L}\sqrt{\frac{\pi m}{2 k_BT}}$

where

$\bar{c}$ is the average molecular speed from the Maxwell–Boltzmann distribution, [L¹ T^-1]
T is the thermodynamic temperature, [θ¹]
μ is the dynamic viscosity, [M¹ L^-1 T^-1]
m is the molecular mass, [M¹]
k_B is the Boltzmann constant, [M¹ L² T^-2 θ^-1]
ρ is the density, [M¹ L^-3].

The dimensionless Mach number can be written:

$\mathit{Ma} = \frac {U_\infty}{c_s}$

where the speed of sound is given by

$c_s=\sqrt{\frac{\gamma R T}{M}}=\sqrt{\frac{\gamma k_BT}{m}}$

where

U_∞ is the freestream speed, [L¹ T^-1]
R is the Universal gas constant, (in SI, 8.314 47215 J K⁻¹ mol⁻¹), [M¹ L² T^-2 θ^-1 'mol'^-1]
M is the molar mass, [M¹ 'mol'^-1]
$\gamma$ is the ratio of specific heats, and is dimensionless.

The dimensionless Reynolds number can be written:

$\mathit{Re} = \frac {\rho U_\infty L}{\mu}.$

Dividing the Mach number by the Reynolds number,

$\frac{Ma}{Re}=\frac{U_\infty \div c_s}{\rho U_\infty L \div \mu }=\frac{\mu }{\rho L c_s}=\frac{\mu }{\rho L \sqrt{\frac{\gamma k_BT}{m}}}=\frac{\mu }{\rho L }\sqrt{\frac{m}{\gamma k_BT}}$

and by multiplying by $\sqrt{\frac{\gamma \pi }{2}}$ ,

$\frac{\mu }{\rho L }\sqrt{\frac{m}{\gamma k_BT}}\sqrt{\frac{\gamma \pi }{2}}=\frac{\mu }{\rho L }\sqrt{\frac{\pi m}{2k_BT}} = \mathit{Kn}$

yields the Knudsen number.
The Mach, Reynolds and Knudsen numbers are therefore related by:

$Kn = \frac{Ma}{Re} \; \sqrt{ \frac{\gamma \pi}{2}}.$

Aplikasi

The Knudsen number is useful for determining whether statistical mechanics or the continuum mechanics formulation of fluid dynamics should be used: If the Knudsen number is near or greater than one, the mean free path of a molecule is comparable to a length scale of the problem, and the continuum assumption of fluid mechanics is no longer a good approximation. In this case statistical methods must be used.

Problems with high Knudsen numbers include the calculation of the motion of a dust particle through the lower atmosphere, or the motion of a satellite through the exosphere. One of the most widely used applications for the Knudsen number is in microfluidics and MEMS device design.

The solution of the flow around an aircraft has a low Knudsen number, making it firmly in the realm of continuum mechanics. Using the Knudsen number an adjustment for Stokes' Law can be used in the Cunningham correction factor, this is a drag force correction due to slip in small particles (i.e. d_p < 5 µm).

Semoga Bermanfaat.

Ucapan Terima Kasih:

Bapak dan Ibu Guru Semasa SMA

Guru dan Dosen di Pendidikan Fisika, FPMIPA Universitas Pendidikan Indonesia

Sumber:

Arip Nurahman Notes

http://en.wikipedia.org/wiki/Knudsen_number

No comments:

Post a Comment

Inspirations Words

Crescat scientia; Vita Excolatur:
"Let knowledge grow from more to more; and so be human life enriched"

"Leadership is not magnetic personality that can just as well be a glib tongue. It is not making friends and influencing people that is flattery. Leadership is lifting a person's vision to higher sights, the raising of a person's performance to a higher standard, the building of a personality beyond its normal limitations."
~Anonymous~

"Jika Hidup ini seumpama rel kereta api dalam eksperimen relativitas, maka pengalaman demi pengalaman yang mengempur kita dari waktu ke waktu adalah cahaya yang melesat-lesat di dalam gerebong di atas rel itu. Relativitasnya berupa seberapa banyak kita dapat mengambil pelajaran dari pengalaman yang melesat-lesat itu, maka analoginya adalah jika pengalaman yang sama dapat menimpa siapa saja, namun sejauh mana dan secepat apa pengalaman itu memberi pelajaran pada seseorang, hasilnya akan berbeda, relatif satu sama lain."
~Andrea H.~

"Anyone who has never made a mistake has never tried anything new."
~Albert Einstein~

"Scientific thought is the common heritage of mankind"
:Pikiran ilmiah adalah warisan umum umat manusia
~Abdus Salam~

"Pengembaraan Pikiran dan Imajinasi Manusia hendaknya menuntun Kita Kepada Keagungan Yang Maha Kuasa"
~Arip~